Neogaean paleomagnetism constraints on the processes at
the core and surface of the Earth
D. M. Pechersky
Wavelet Analysis
Fourier analysis is commonly used to reveal periodicities in
a time series. However, formal application of
Fourier analysis to the geomagnetic field can produce various artifacts,
false periods, etc. This problem can be
solved through the application of wavelet analysis which compares
a signal studied with a finite wave train
rather than an infinite sinusoid employed in the Fourier analysis.
The geomagnetic polarity time scale is
described by a step function whose Fourier spectrum contains
numerous multiple harmonics, whereas the
wavelet analysis does not produce multiple harmonics and is
efficient in studies of spectral properties of
aperiodic signals [Holschneider, 1995].
Wavelets are a family of oscillating self-similar functions
varying in scale that are localized in both physical
and Fourier spaces. Wavelet analysis allows one to study spectrally
nonstationary processes, examine the
phase behavior of components of a quasi-periodic process,
and estimate its energy characteristics. As
compared with the Fourier analysis, resulting spectra are smoother
and free from multiple and combination
frequencies. Another advantage of the wavelet analysis
is its applicability to time series with missing
observations [Galyagin and Frik, 1996].
The choice of a specific wavelet depends on the purposes of analysis.
In our case [Galyagin et al., 1999], the
Morle and "Mexican hat" wavelets were employed.
The wavelet transformation transfers a function of one
variable
t into the plane of two variables
t and
a.
Here,
t specifies the wavelet center position on the
time axis, and the parameter
a characterizes the time scale
of oscillations and coincides with their period if
the Morle wavelet is used. An analogue of the Fourier spectrum
is the so-called integral wavelet spectrum
obtained by integration of the squared wavelet modulus along the time axis.
Wavelet analysis of the reversal frequency in the Neogaea.
 Periods of the integral wavelet spectrum
F(t) (15, 30, 50-60, 70, 100-110, 130, 180, 220, 280, and 390 Myr)
are essentially the same as those
previously obtained
[Marzocchi and Mulargia, 1992;
McElhinny, 1971;
Merrill and McElhinny, 1983;
Pechersky and Nechaeva, 1988].
As distinct from other methods of spectral analysis, wavelet data provide
time evolution of the spectrum. We consider the Neogaean
evolution of the wavelet spectrum (Figure 4)
[Galyagin et al., 1999].
First, the Vendian-Phanerozoic and Riphean patterns are drastically different.
Second, an oscillation with a period of about 400 Myr
is recognizable throughout the Vendian-Phanerozoic
interval; this period decreases from more than 450 Myr
in the Vendian to less than 400 Myr in the Late
Cenozoic. The lifetime of remaining oscillations with periods of 70 to 200 Myr
is much shorter. Thus,
oscillations with periods of 90 and 180 Myr
are traceable from 550 to 200 Ma, when both periods gradually
decreased from 200 to 170 Myr and from 100 to 90 Myr, respectively.
A 130-Myr period is stable and exists
from 180 Ma to the present time, and its resolution markedly improves
toward the Cenozoic; a 70-Myr period
exists from 160 Ma to the present time and decreases from 70 to 55 Myr.
Third, the Phanerozoic and Early
Riphean exhibit a series of "pulses" (approximately at 1650, 540, 370,
and 160 Ma) at the lower boundary of
the wavelet plane, which indicates their characteristic times to be about 30 Myr.
Periods of the integral wavelet spectrum
F(t) (15, 30, 50-60, 70, 100-110, 130, 180, 220, 280, and 390 Myr)
are essentially the same as those
previously obtained
[Marzocchi and Mulargia, 1992;
McElhinny, 1971;
Merrill and McElhinny, 1983;
Pechersky and Nechaeva, 1988].
As distinct from other methods of spectral analysis, wavelet data provide
time evolution of the spectrum. We consider the Neogaean
evolution of the wavelet spectrum (Figure 4)
[Galyagin et al., 1999].
First, the Vendian-Phanerozoic and Riphean patterns are drastically different.
Second, an oscillation with a period of about 400 Myr
is recognizable throughout the Vendian-Phanerozoic
interval; this period decreases from more than 450 Myr
in the Vendian to less than 400 Myr in the Late
Cenozoic. The lifetime of remaining oscillations with periods of 70 to 200 Myr
is much shorter. Thus,
oscillations with periods of 90 and 180 Myr
are traceable from 550 to 200 Ma, when both periods gradually
decreased from 200 to 170 Myr and from 100 to 90 Myr, respectively.
A 130-Myr period is stable and exists
from 180 Ma to the present time, and its resolution markedly improves
toward the Cenozoic; a 70-Myr period
exists from 160 Ma to the present time and decreases from 70 to 55 Myr.
Third, the Phanerozoic and Early
Riphean exhibit a series of "pulses" (approximately at 1650, 540, 370,
and 160 Ma) at the lower boundary of
the wavelet plane, which indicates their characteristic times to be about 30 Myr.
The stability of the inferred results is corroborated by their
similarity for wavelet processing procedures with
smoothing windows varying from 5 to 30 Myr.
Wavelet analysis of the geomagnetic polarity time scale.
It is interesting to apply the wavelet analysis to
the polarity scale proper
[Galyagin et al., 1998, 1999].
The integral wavelet spectrum is relatively smooth and
exhibits one pronounced peak ( a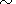 180 Myr) and a number of weak peaks
at 12, 23, 43, 60, 100, 180, 300, and 600 Myr
[Galyagin et al., 1999].
The picture on the wavelet plane is considerably different from the
reversal frequency spectrum (Figure 4).
The oscillation with a 180-Myr period exists over the interval 1450-850 Ma.
The period is 160 Myr in the middle of the interval and increases
to 180-200 Myr at its ends. In the
Phanerozoic, this pattern is changed by a rather stable oscillation,
with its period gradually increasing from
250 to 300 Myr in the interval 450-100 Ma.
The remaining "periods" observed in the integral spectrum are
due to local events (pulses). Three intervals are recognized:
(a) Vendian-Phanerozoic (630-0 Ma), includes
five pulses, with average spacing between them being 125 Myr;
(b) middle Middle Riphean-middle Late
Riphean (1260-860 Ma), five pulses, average spacing of 80 Myr;
and (c) Early-Middle Riphean (1670-1260 Ma),
three pulses, average spacing of 140 Myr.
A "quiescence" interval is confidently defined between 850 and 650 Ma
(Figure 4).
180 Myr) and a number of weak peaks
at 12, 23, 43, 60, 100, 180, 300, and 600 Myr
[Galyagin et al., 1999].
The picture on the wavelet plane is considerably different from the
reversal frequency spectrum (Figure 4).
The oscillation with a 180-Myr period exists over the interval 1450-850 Ma.
The period is 160 Myr in the middle of the interval and increases
to 180-200 Myr at its ends. In the
Phanerozoic, this pattern is changed by a rather stable oscillation,
with its period gradually increasing from
250 to 300 Myr in the interval 450-100 Ma.
The remaining "periods" observed in the integral spectrum are
due to local events (pulses). Three intervals are recognized:
(a) Vendian-Phanerozoic (630-0 Ma), includes
five pulses, with average spacing between them being 125 Myr;
(b) middle Middle Riphean-middle Late
Riphean (1260-860 Ma), five pulses, average spacing of 80 Myr;
and (c) Early-Middle Riphean (1670-1260 Ma),
three pulses, average spacing of 140 Myr.
A "quiescence" interval is confidently defined between 850 and 650 Ma
(Figure 4).
Overall, wavelet planes of the polarity scale and reversal frequency
(in essence, entirely different entities)
yield similar evolution patterns of geomagnetic field in the Phanerozoic
and Precambrian [Galyagin et al., 1999].
Summary Amplitude of Paleovariations in the Geomagnetic
Field Direction.
The geomagnetic polarity time scale yields evidence
of the field sign, i.e. maximum changes of the field
direction. The paleomagnetic database
[McElhinny and Lock, 1990, 1993]
provides the possibility of
estimating the summary amplitude of paleovariations in the
field direction, amounting to a few tens of
degrees. Theoretically, the summary amplitude is defined by
the standard angular deviation
S=81/K1/2 [Fisher et al., 1987;
Khramov et al., 1982;
Merrill and McElhinny, 1983],
where
K is the precision parameter of individual vector directions
for their normal distribution on sphere. The
S value was
determined in this way for the whole Neogaea
[Pechersky, 1996, 1997].
Index of paleomagnetic reliability.
Each paleomagnetic determination of the paleomagnetic database is
characterized by its index of paleomagnetic reliability (IPR) varying
from 0 to 1 and depending on the number
of samples involved in a given determination, precision parameter
of paleomagnetic directions, availability of
thermal and alternating field demagnetization data, paleomagnetic
reliability tests (baked contact, pebble,
fold, and reversal tests), quality of laboratory paleomagnetic measurements
and their processing, and age
determinations of rocks and stable component of natural remanent magnetization
(NRM). Age determination
was shown to have the greatest effect on the IPR value
(particularly for Precambrian rocks) and considerably
increase the scatter in
S values close in age.
The IPR value was taken as a weight in the calculation of
S means for each 10-Myr intervals
[Pechersky, 1996, 1997].
Weighted means of
S from volcanic and sedimentary rocks younger than 10 Ma
do not differ
on a significant level; decreasing IPR results in an increase in
DS from 3-4o at IPR
> 0.6 to
8o at IPR
 0.2.
Therefore, low-IPR
S means may be considered reliable,
which is especially important in the case of
Precambrian determinations dominated by IPR
0.2.
Therefore, low-IPR
S means may be considered reliable,
which is especially important in the case of
Precambrian determinations dominated by IPR
 0.2.
0.2.
Rock types.
All rocks are subdivided
into four groups according to their NRM origin: (a) volcanic rocks
with the most probable primary thermal remanent magnetization (TRM);
in the context of our study, the lava
cooling and related acquisition of geomagnetic direction may be considered
instantaneous; (b) intrusive rocks
with probably dominating primary TRM; however, due to slow cooling
of large bodies, variations may be
averaged in a collection and even in an individual sample;
(c) sedimentary rocks; they may preserve
information about primary detrital remanent magnetization and exhibit marked
averaging of variations within
a sample due to slow deposition of sediments, postsedimentary processes,
and early diagenesis; (d) "metamorphic" rocks; these are rocks
that experienced various alterations, including the acquisition of
secondary, often postfolding, crystallization or chemical remanent
magnetization; acquisition time of ancient
magnetization in this rock group can be long, and as a result the scatter
in
S values is largest. Evidently,
metamorphism affects all groups of rocks, particularly ancient ones,
which gives rise to a higher scatter in
S values and thereby affects both the magnetization age and
their relation to the variation amplitude.
Weighted averages of
S insignificantly differ in young volcanic,
intrusive, and sedimentary rocks, and
relative variations in
S, averaged over 10-Myr age intervals
in the Phanerozoic and Precambrian are also similar
[Pechersky, 1997].
In view of this similarity, data on all types of rocks are grouped
in accordance with
their IPR weight (Figure 1d). Information is irregularly distributed in time:
data from the Phanerozoic are
more representative, whereas Precambrian determinations are fewer,
and their quality is worse
(determinations with IPR
 0.2 are predominant)
[Pechersky, 1997].
0.2 are predominant)
[Pechersky, 1997].
Influence of geomagnetic reversals.
To include the possible effect of transitional reversal zones,
collections with a constant polarity
(S0) and those with both polarities
(Sm) were separately
analyzed. The probability of finding transitional-zone samples
in the mixed-polarity collections was higher.
Correspondingly, the
S value from mixed-polarity collections of coeval
rocks of one group is on average
markedly higher than from single-polarity collections. This fact was
accounted for through division by
Sm/S0 averaged over rocks of a given age from
a given group,
which reduced the scatter in data but did not affect the
general behavior of
S, and the Precambrian pattern became better resolved
[Pechersky, 1997].
Latitude effect.
To analyze the dependence of
S on the determination latitude of paleomagnetic
direction, the deviation of paleomagnetic latitude from its actual
value should be first found. Possible
underestimation of paleomagnetic latitude was analyzed using paleomagnetic
data over the past 10 Myr, when
the true latitude of the stable NRM component acquisition, insignificantly
disturbed by lateral movement of crustal blocks, is known
[Pechersky, 1996].
Mean paleolatitudes, calculated from
10o intervals of the true
latitude of sampling sites for both volcanic and sedimentary rocks from
mixed- and single-polarity collections,
deviate from the true latitude by no more than
10o.
Thus, the paleolatitude underestimation is on average insignificant.
 We consider the latitude dependence of
S for two main states
of the geomagnetic field: (a) stable normal or
reversed polarity, and (b) frequent polarity reversals
[Pechersky, 1996, 1997]. Averaging
data over age
intervals of the stable field (Figure 5) yields the inverse
latitude dependence of
S close to the dipole field
model of secular variations, secular variation amplitude of
the present field observed in the northern hemisphere
[Kono and Tanaka, 1995;
McElhinny and McFadden, 1997;
Pesonen et al., 1994;
Yanovskii, 1978],
and paleovariation amplitude in the Permian and Carboniferous,
when the field was stable
[Khramov et al., 1982].
This dependence is not observed during the high reversal frequency intervals
(Figure 5d),
which
allows the following conclusions to be drawn. (1) The latitude dependence
of
S implies that its average
value yields adequate constraints on the summary amplitude of paleovariations
in the field direction. (2) Field
generation conditions in the 1700-Ma interval were similar
in the periods of stable (normal and reversed)
polarity. (3) The summary amplitude of direction variations did not depend
on latitude in the high
reversal frequency intervals. The mean amplitude of the unstable
field behavior ( S
We consider the latitude dependence of
S for two main states
of the geomagnetic field: (a) stable normal or
reversed polarity, and (b) frequent polarity reversals
[Pechersky, 1996, 1997]. Averaging
data over age
intervals of the stable field (Figure 5) yields the inverse
latitude dependence of
S close to the dipole field
model of secular variations, secular variation amplitude of
the present field observed in the northern hemisphere
[Kono and Tanaka, 1995;
McElhinny and McFadden, 1997;
Pesonen et al., 1994;
Yanovskii, 1978],
and paleovariation amplitude in the Permian and Carboniferous,
when the field was stable
[Khramov et al., 1982].
This dependence is not observed during the high reversal frequency intervals
(Figure 5d),
which
allows the following conclusions to be drawn. (1) The latitude dependence
of
S implies that its average
value yields adequate constraints on the summary amplitude of paleovariations
in the field direction. (2) Field
generation conditions in the 1700-Ma interval were similar
in the periods of stable (normal and reversed)
polarity. (3) The summary amplitude of direction variations did not depend
on latitude in the high
reversal frequency intervals. The mean amplitude of the unstable
field behavior ( S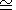 15o, Figure 5d) does
not differ from the mean amplitude of the stable one near the equator
(Figure 5i); therefore, the
S independence of latitude is not due to an increase in the variation
amplitude that would mask
S variation
with latitude, but it is a regularity inherent in a specific generation mode
of the geomagnetic field. The
example of secular variations in the present field direction has shown that,
first, their latitude dependence is
markedly different in the northern and southern hemispheres
[McFadden et al., 1988;
Merrill and McElhinny, 1983;
Pesonen et al., 1994];
second, the secular variation amplitude depends not only on
latitude but also, to large degree, on longitude
[Shibuya et al., 1995;
Tsunakawa, 1988];
and, third, the field that existed at the
core surface during the past 300 years includes, in addition
to the dipole part, a combination of stable
constant, immobile fluctuating, and drifting components
[Gubbins, 1987],
which markedly smooth the average latitude dependence.
The above indicates that the amplitude of secular variations
in the field direction
is dominated by a nondipole component which strongly varies
at the Earth's surface during epochs of unstable
geomagnetic field behavior, whereas during stable field periods
the nondipole field variations are regular and
symmetrical and, as a result, evidently depend on latitude.
The two types of field behavior are also
recognizable from the analysis of geomagnetic polarity over the past 1700 Myr,
providing an independent
argument for the result derived from the study of the variation amplitude.
The accuracy and representativity of
data are still insufficient for the analysis of intermediate field
states between the two regimes considered
above, transition time between those, and stability time intervals
of each regime. The field stability intervals
are likely to exist during very short times, alternating with
and superimposing on one another
[Pesonen et al., 1994].
15o, Figure 5d) does
not differ from the mean amplitude of the stable one near the equator
(Figure 5i); therefore, the
S independence of latitude is not due to an increase in the variation
amplitude that would mask
S variation
with latitude, but it is a regularity inherent in a specific generation mode
of the geomagnetic field. The
example of secular variations in the present field direction has shown that,
first, their latitude dependence is
markedly different in the northern and southern hemispheres
[McFadden et al., 1988;
Merrill and McElhinny, 1983;
Pesonen et al., 1994];
second, the secular variation amplitude depends not only on
latitude but also, to large degree, on longitude
[Shibuya et al., 1995;
Tsunakawa, 1988];
and, third, the field that existed at the
core surface during the past 300 years includes, in addition
to the dipole part, a combination of stable
constant, immobile fluctuating, and drifting components
[Gubbins, 1987],
which markedly smooth the average latitude dependence.
The above indicates that the amplitude of secular variations
in the field direction
is dominated by a nondipole component which strongly varies
at the Earth's surface during epochs of unstable
geomagnetic field behavior, whereas during stable field periods
the nondipole field variations are regular and
symmetrical and, as a result, evidently depend on latitude.
The two types of field behavior are also
recognizable from the analysis of geomagnetic polarity over the past 1700 Myr,
providing an independent
argument for the result derived from the study of the variation amplitude.
The accuracy and representativity of
data are still insufficient for the analysis of intermediate field
states between the two regimes considered
above, transition time between those, and stability time intervals
of each regime. The field stability intervals
are likely to exist during very short times, alternating with
and superimposing on one another
[Pesonen et al., 1994].
Thus, similar to the geomagnetic polarity behavior (Figure 1d),
two intervals, Phanerozoic and Precambrian,
differing in the type of variations in
S are recognized, and the
boundary between them lies at about 600 Ma.
This may be explained in part by the amount and quality of data,
but the characteristic features discussed
above suggest that these two types of polarity behavior are
likely to have actually existed. Irrespective of
paleolatitude, the value of
S very smoothly increases from
13o to
16o in a 1450-450-Ma interval and
afterward gradually decreases to
12o until presently. High-frequency
S fluctuations within
1-4o occur
against the background of this smooth variation in the amplitude
of paleovariations.
Wavelet analysis of the summary amplitude of field direction
variations [Galyagin et al., 1999].
The smooth integral wavelet spectrum of the
S time series exhibits weak peaks
at 45, 80, 160, 300, 450, and
about 1000 Myr. As is evident from the wavelet plane (Figure 4),
these periods are local maximums. The
pattern is rather uniform at
a> 100 Myr.
The difference between the Phanerozoic and Precambrian
generation modes is obvious at characteristic times smaller than 100 Myr:
an interval of more intense
Precambrian variations is observed at these times.
Variation in the Paleointensity (Modulus of the Field Intensity)
The absolute value of paleointensity is mostly determined by using
heating methods (Thellier, Wilson-Burakov, van Zijl, Shaw, and others
[Khramov et al., 1982;
Merrill and McElhinny, 1983;
Pechersky, 1985]).
The Thellier method with controllable mineral alterations is believed
to be most reliable. In addition to
determinations of the paleointensity
Ha and related estimates of the magnetic dipole moment (DM)
incorporated in the Database
[Tanaka and Kono, 1994],
we used data on the Paleozoic and Precambrian that
were not included in this database
[Harcombe-Smee et al., 1994;
Mikhailova et al., 1994, 1996;
Oppenheim et al., 1994;
Pavlov et al., 1992;
Starunov et al., 1996;
Thomas, 1993;
Thomas and Piper, 1995;
Thomas et al., 1995;
Ueno, 1995].
The
Ha and DM determinations are distributed very irregularly in time.
Thus, in many intervals
occasionally reaching a length of 100 Myr,
the determinations are absent at all (Figure 1e)
[Pechersky, 1998].
Coverage of a 400-0-Ma interval (1058 determinations) is on the whole
satisfactory, whereas only 89 determinations are available for
the Early Paleozoic-Precambrian. 681 of 1063 determinations from the
Phanerozoic and 83 of 84 in the Riphean were made by using the Thellier method
[Pechersky, 1998].
The values of paleointensity and related DM vary within a wide range,
from
< 5 to
> 100
m T (Figures 1e-1g).
(The majority of
Ha> 100
m T values were obtained with the
help of the Shaw method!) The
scatter (standard deviation) in the
Ha and DM
determinations close in age was taken as a characteristic of
the paleointensity variation amplitude.
Reliability index of paleointensity determination.
Similar to the analysis of data on paleomagnetic
directions used for estimation of the amplitude of paleovariations,
reliability indexes of paleointensity
determinations (RIPs), ranging from 0 to 1, are introduced.
The maximum RIP = 1 characterizes samples
from baking zones and rapidly cooling lavas; maximum RIPs of 1, 0.6, 0.6,
and 0.5 are given by the methods
of Thellier, Shaw, Wilson-Burakov, and van Zijl, respectively;
and RIP values of not more than 0.2
characterize other methods and paleointensity estimates obtained
from relative values of thermoremanent
magnetization (TRM) created in laboratory in a given external magnetic
field and natural remanent
magnetization (NRM). If data on paleomagnetic direction are unavailable,
a value of 0.3 is subtracted from the
pertinent RIP. RIP values reduced by 0.2-0.3 are commonly adopted
for determinations made before 1970
and, finally, lower RIP values are assigned to less accurately dated
determinations. Most authors assume that
ages of rocks and stable natural remanence component coincide,
provided the component is a primary TRM, the Database
[Tanaka and Kono, 1994]
provides very little information about the validity of the primary
thermal origin of remanence. Calculation of mean paleointensities includes
a RIP as a weight of each determination.
The majority of determinations have RIP
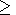 0.5
(RIPs are close to unity for many of those) mostly due to
numerous Thellier determinations from baked rocks, performed by
G. M. Solodovnikov and covering a 400-0-Ma interval.
RIP does not exceed 0.2 in the age intervals 70-80, 170-180, 220-230,
and 360-370 Ma; most
Riphean determinations have RIP = 0.4-0.5 mainly due to lacking
information about paleomagnetic
directions and inaccuracy of datings [Pechersky, 1998].
0.5
(RIPs are close to unity for many of those) mostly due to
numerous Thellier determinations from baked rocks, performed by
G. M. Solodovnikov and covering a 400-0-Ma interval.
RIP does not exceed 0.2 in the age intervals 70-80, 170-180, 220-230,
and 360-370 Ma; most
Riphean determinations have RIP = 0.4-0.5 mainly due to lacking
information about paleomagnetic
directions and inaccuracy of datings [Pechersky, 1998].
The influence of geomagnetic reversals.
Paleointensity is known to considerably decrease during
geomagnetic reversals and excursions, but we are not interested
in the field behavior during reversals. For this
reason we did not consider Database determinations that were
attributed by their authors to reversal zones, as
well as abnormally low
Ha values whose directions are untypical
of their time and site.
Latitude effect.
 A close linear relation between
Ha and DM (Figure 6)
[Pechersky, 1998] reflects
obvious predominance of the dipole component in
Ha. The
Ha value changes across the
Ha (DM band by about 35%,
yielding variation in the present dipole field intensity from equator to pole.
The band length which reflects the amplitude of variations in both
Ha and DM considerably overlaps the possible
latitude dependence of
Ha.
A close linear relation between
Ha and DM (Figure 6)
[Pechersky, 1998] reflects
obvious predominance of the dipole component in
Ha. The
Ha value changes across the
Ha (DM band by about 35%,
yielding variation in the present dipole field intensity from equator to pole.
The band length which reflects the amplitude of variations in both
Ha and DM considerably overlaps the possible
latitude dependence of
Ha.
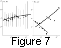 I consider the latitude dependence of paleointensity for two cases
of geomagnetic field regime: (1) unstable,
with frequent reversals, and (2) stable, with very rare reversals.
The time intervals 45-0 Ma and 310-260 Ma
will be examined where paleointensity data are sufficiently representative
and cover a wide range of
paleolatitudes. In both cases, irrespective of the reversal regime,
Ha averages over latitude intervals are
close to the line representing the latitude dependence of field
intensity of the central axial dipole (Figure 7a);
therefore, the paleointensity is close to the central axial dipole
field irrespective of the reversal regime. The
closeness of the DM to the central axial dipole is substantiated by
the comparison of average DMs in the
southern and northern hemispheres in the age interval 7-0 Ma.
Such an interval is chosen because it is the
only one in which the numbers of DM determinations in the southern
(56 collections) and northern (127 collections)
are more or less comparable and cover
a sufficiently wide interval of latitudes. Average DMs in
the northern and southern hemispheres are differ insignificantly:
(7.4
I consider the latitude dependence of paleointensity for two cases
of geomagnetic field regime: (1) unstable,
with frequent reversals, and (2) stable, with very rare reversals.
The time intervals 45-0 Ma and 310-260 Ma
will be examined where paleointensity data are sufficiently representative
and cover a wide range of
paleolatitudes. In both cases, irrespective of the reversal regime,
Ha averages over latitude intervals are
close to the line representing the latitude dependence of field
intensity of the central axial dipole (Figure 7a);
therefore, the paleointensity is close to the central axial dipole
field irrespective of the reversal regime. The
closeness of the DM to the central axial dipole is substantiated by
the comparison of average DMs in the
southern and northern hemispheres in the age interval 7-0 Ma.
Such an interval is chosen because it is the
only one in which the numbers of DM determinations in the southern
(56 collections) and northern (127 collections)
are more or less comparable and cover
a sufficiently wide interval of latitudes. Average DMs in
the northern and southern hemispheres are differ insignificantly:
(7.4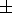 3.6)
3.6) 1022 A m
2 (RIP=0.65)
and
(8.5
1022 A m
2 (RIP=0.65)
and
(8.5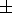 2.7)
2.7) 1022 A m
2 (RIP=0.77), respectively.
To verify stability of these results, the age
interval was divided into the intervals 0.9-0 and 7-1 Ma,
which gave nearly the same values
[Pechersky, 1998].
Thus, one may state that the geomagnetic field is close to that
of a central axial dipole.
1022 A m
2 (RIP=0.77), respectively.
To verify stability of these results, the age
interval was divided into the intervals 0.9-0 and 7-1 Ma,
which gave nearly the same values
[Pechersky, 1998].
Thus, one may state that the geomagnetic field is close to that
of a central axial dipole.
The latitude dependence of
Ha variations is quite different (Figure 7b):
the high reversal frequency period
is characterized by an increase of the variation amplitude with latitude,
which does not agree with the dipole
field behavior, whereas the amplitude decreases with latitude,
in accordance with the dipole field, during the stable field period.
Smoothing.
To reduce the scatter in data connected
with dating uncertainties and systematic errors, all
results were subdivided into 10-Myr intervals in which
Ha and DM averages and standard deviations
dH were calculated, with weights taken in accordance with RIP indexes.
The 10-Myr averages were then
smoothed both with and without weighting. Smoothing windows of 30 Myr
and more in length and a step of 10 Myr were used
[Pechersky, 1998].
Wavelet analysis of paleointensity.
In contrast to the geomagnetic polarity scale, the time series
of the
intensity modulus includes noticeable breaks (Figure 1)
[Pechersky, 1998].
A specific wavelet analysis
procedure was developed to analyzed such series
[Galyagin and Frik, 1996;
Galyagin et al., 1999].
The integral spectrum of
Ha exhibits peaks of 45, 140, 260, and 560 Myr.
The wavelet plane of
Ha (Figure 1)
[Galyagin et al., 1999]
differs from those discussed above. Only two oscillations with relatively long
periods of 230-260 Myr (from about 500 Ma until present time) and 135 Myr
(approximately from 1200 to
1000 Ma) are more or less reliably recognized, and a series of local
maximums with periods of 30 to 80 Myr is
additionally observed. Two areas of 1600-1450 and 850-450 Ma,
clearly observed on the plane, are related in
part to lacking data (Figure 1e); on the other hand, they resemble
similar areas of other field characteristics,
not related to lacking data (Figure 1). This mostly concerns the
boundary region between the Riphean and
Phanerozoic (circa 850-450 Ma).
Wavelet analysis of paleointensity variations.
To analyze the paleointensity variation, I consider the time
behavior of the standard deviation
dH and ratio
dH/Ha.
The integral spectrum of
dH is very close to
the
Ha spectrum and has only one additional peak yielding
a period of 60 Myr. The spectrum
dH/Ha is
somewhat different and exhibits peaks with 40, 100, 180, 320, and 500 Myr.
Like integral spectra, the wavelet
spectra of
Ha and
dH are on the whole similar
[Galyagin et al., 1999].
Thus, the higher the intensity of
an oscillation, the stronger its variation. The wavelet plane of
dH/Ha has generally the same pattern as those of
Ha and
dH.
The general pattern of paleointensity behavior.
The above data on the paleointensity behavior reveal three
tendencies: (1) The
Ha and
dH scatter within comparatively
narrow time limits is likely to reflect the
paleointensity variation amplitude. (2) Relatively smooth long-period
cyclic variations in
Ha and DM are
observed (with periods ranging from several tens to several hundreds
of millions of years). (3) The average
paleointensity level in Precambrian (DM averages
6.5 1022 A m
2 )
is higher than in Phanerozoic (DM
averages
4.8
1022 A m
2 )
is higher than in Phanerozoic (DM
averages
4.8 1022 A m
2 ); the Riphean is characterized
by a general drop in paleointensity, and its
general increase is observed in the Phanerozoic.
1022 A m
2 ); the Riphean is characterized
by a general drop in paleointensity, and its
general increase is observed in the Phanerozoic.
Main Features of the Geomagnetic Field Behavior in the
Neogaea
1. The Neogaea is evidently dominated by the reversed geomagnetic polarity,
but the normal polarity
percentage increases, against the background of marked fluctuations,
from the Early Paleozoic to the present
time; the whole Phanerozoic is a transitional, unstable-polarity interval.
This instability appears as a marked
increase in reversal frequency and the shortening of single-polarity magnetic
zones. The mean reversal
frequency is less than 1 reversal every 10 Myr in the Precambrian
(magnetic zones 1 to 100 Myr long are
predominant), about 6 reversals every 10 Myr in the
Paleozoic (magnetic zones 0.5 to 5 Myr long), over 8
reversals every 10 Myr in the Mesozoic (0.2 to 2.5 Myr),
and about 30 reversals every 10 Myr in the Cenozoic
(0.05 to 1 Myr), the frequency increasing from 12 per 10 Myr
in the Early Cenozoic to 43 in the last 10-Myr
interval. The aforementioned asymmetry of the field, as well as
the regular distributions of its reversal
frequency and lengths of constant-polarity intervals indicate that
at least two field generation modes existed
over the past 1700 Myr. The first mode was typical of
the Precambrian
and Paleozoic, when long stable-field
intervals of mostly reversed polarity prevailed; the second mode
characterized by frequent reversals was
typical of Mesozoic and especially Cenozoic. Activities of both modes
largely overlapped in time. Long stable
field intervals of constant polarity were rather uniformly distributed
in the Neogaea: their centers (Figure 1a, b) at about 1680, 1520,, 1360,
1150, 1100, 900, 700, 630, 470, 290, and 100 Ma are spaced by 160-200 Myr
except for two anomalies between 1150 and 1100 Ma and between 700 and 630 Ma,
whereas the reversal
frequency between these intervals considerably increases in the Phanerozoic.
The reversal sequence is fractal, with the dimensions
d 0.5-0.6
and
0.5-0.6
and
 0.9, i.e., has the property of self-similarity
of
large-scale processes in accordance with the observed reversal distribution:
alternation of high
reversal frequency zones with fairly long intervals of rare reversals.
On the other hand, their distribution
within intervals of high reversal frequency is nearly chaotic
(d<0.6).
0.9, i.e., has the property of self-similarity
of
large-scale processes in accordance with the observed reversal distribution:
alternation of high
reversal frequency zones with fairly long intervals of rare reversals.
On the other hand, their distribution
within intervals of high reversal frequency is nearly chaotic
(d<0.6).
2. The summary amplitude behavior of field direction paleovariations
is on the whole characterized by the
same regularities as the reversal frequency variations;
the Riphean amplitudes vary between
10o and
14o and occasionally reach
20o ; their general level weakly
rises, whereas an inverse situation is observed in
the Phanerozoic: against the background of weak fluctuations,
S smoothly falls from
18o in the
Vendian to
11o in the Cretaceous. The analysis of latitude
dependence of
S from three main states of the
geomagnetic field (stably normal polarity, stably reversed polarity,
and frequent reversals) leads to the
following conclusions. (a) The variation in
S with latitude implies
that the paleovariations in the summary
amplitude of geomagnetic field, prevailing in the Precambrian and Paleozoic,
are consistent with a central
axial dipole, and (b) this amplitude was on average independent
of the paleolatitude in high reversal
frequency zones. Furthermore, average amplitudes of unstable and stable
regimes virtually coinciding, the
invariability of
S with latitude is evidence of a different mode
of geomagnetic field generation.
Mean paleolatitudes calculated from paleomagnetic inclinations provided
by collections of young volcanic and
sedimentary rocks of single or mixed polarity differ from the true latitude
by less than
10o, which implies
that, on average, the paleolatitude is not overly underestimated.
3. Three tendencies are recognizable in the intensity behavior
during the Neogaea:
(a) The scatter in
Ha and DM (standard deviation
dH )
within relatively narrow time limits is likely to
reflect the amplitude of intensity paleovariations; the
dH/Ha value appears to correlate with intervals of
predominant reversed polarity (especially noticeable in the Riphean)
and with reversal frequency.
(b) Relatively smooth long-period cyclic variations in
Ha and DM are observed (over times of few tens to
few hundreds of millions of years). Correlated extremums being nearly
as frequent as anticorrelated ones, the
time distribution of extremums of both types is rather chaotic except
for the correlation between the DM and
polarity asymmetry: correlation of extremums is almost everywhere normal
in the Phanerozoic and inverse in
the Riphean. The
dH and
dH/Ha values correlate with
the polarity asymmetry and reversal frequency
F,
and the polarity asymmetry is anticorrelated with
F and
S in the Riphean
(the correlation is mostly
positive in the Mesozoic and Cenozoic). Most noticeable is the correlation,
observed throughout the Neogaea,
between the lower amplitude relative variation of the paleointensity
dH/Ha and Neogaean intervals of lower
frequency or absence of reversals (Figure 1).
(c) The mean level of paleointensity in the Precambrian
(DM =
6.5 1022 A m
2 ) was higher than in the
Phanerozoic (DM =
4.8
1022 A m
2 ) was higher than in the
Phanerozoic (DM =
4.8 1022 A m
2 ); the Riphean
is characterized by a general drop in the
paleointensity, and the Phanerozoic, by its rise.
The general Phanerozoic rise in paleointensity correlates with
an increase in the summary amplitude of field direction paleovariations,
in the reversal frequency, and in the
percentage of normal polarity. On the whole, higher paleointensity
and its smooth decrease in the Riphean
correlate with the evident predominance of reversed polarity and very
low reversal frequency in the same period.
1022 A m
2 ); the Riphean
is characterized by a general drop in the
paleointensity, and the Phanerozoic, by its rise.
The general Phanerozoic rise in paleointensity correlates with
an increase in the summary amplitude of field direction paleovariations,
in the reversal frequency, and in the
percentage of normal polarity. On the whole, higher paleointensity
and its smooth decrease in the Riphean
correlate with the evident predominance of reversed polarity and very
low reversal frequency in the same period.
The paleointensity is close to the field of a central axial dipole
irrespective of the reversal regime, whereas
variations in
Ha show quite different latitude dependences:
with increasing latitude, the amplitude of
paleointensity variations increases in the case of the high reversal
frequency regime and decreases in intervals of a stable field.
4. The Riphean trend of all geomagnetic characteristics changes
to the Phanerozoic one at about 600 Ma.
5. Datasets providing constraints on the reversal frequency and polarity
asymmetry, on
dH/Ha and DM, and on
S are virtually independent (this is especially true for the first two sets).
This is an argument confirming the
validity of the correlations between these parameters.
6. Wavelet analysis of the reversal frequency, field
sign, paleointensity,
variations in the field direction and
intensity, and their Neogaean evolution indicated that the geomagnetic
field was generally unstable, i.e., its
evolution involved no periodical processes. The following facts support
this conclusion (Figure 4)
[Galyagin et al., 1999].
(a) A marked difference between the general patterns of wavelet spectra of
all geomagnetic parameters
considered above is observed. For example, if the reversal frequency
F is markedly more pronounced in the
Phanerozoic, the summary amplitude of variations in the field direction
S,
as well as change in the polarity
sign
P, are considerably more "active" in the Precambrian.
(b) The majority of derived periods are represented by short "events"
whose length amounts to one or two full
oscillations. Only four of about one hundred of such events include three
to five oscillations of a given period;
these are the oscillations of the reversal frequency
F at a period of
100-110 Myr in the age interval from
550 to 160 Ma, oscillations of the single-polarity magnetic zone length
at a period of 160-200 Myr observed
from 1500 to 850 Ma, oscillations of relative variation in paleointensity
dH/Ha at a period of 90-100 from 450
to 100 Ma, and oscillations of the summary amplitude of variations
in the field direction
S at a period of 75-90 Myr from 1450 to 1150 Ma.
(c) Oscillations of various characteristics at close
periods are commonly
asynchronous. A Riphean
interval of 1500 to 800 Ma is noticeable in which oscillations of field
sign and paleointensity variations with
close periods of 140-180 Myr are grouped. In the Vendian, they continue
as oscillations of the same
characteristics and reversal frequency at a period of about 220-300 Myr
(Figure 4).
Moreover, oscillations of
F,
P,
dH/Ha, and
S at a period of about 100 Myr
are grouped in the Phanerozoic interval between 500
and 100 Ma. The remaining preferred intervals of "periods",
without regard to the occurrence time of related
oscillations, are as follows: 40-45 Myr
( F,
P,
Ha,
dH,
dH/Ha,
S ); 60-70 Myr
( F,
P,
dH ); 80-100 ( F,
dH/Ha,
S ); 130-140 Myr
( F,
Ha,
dH ); 160-180 Myr ( F,
P,
dH/Ha,
S );
250-260 Myr ( Ha,
dH ): 300-320 Myr ( dH/Ha,
S );
and 500-600 Myr ( P,
Ha,
dH,
dH/Ha ).
(d) Oscillation periods often smoothly vary with time
(Figure 4),
which is largely responsible for wide ranges
of periods indicated in item "c". Most periods decrease with time
concordantly with the general acceleration of
the process; thus the
F period falls from 100 to 85 Myr over a
470-160-Ma interval and from 70 to 60 Myr
over a 150-0-Ma interval, and the
S period falls from 90 to 70 Myr
during the time from 1500 to 1100 Ma.
The cases of an increase in periods, consistent with the slowing-down
of the process are less frequent. For
example, the
P and
dH/Ha periods increase, respectively,
from 200 to 280 Myr and from 80 to 100 Myr over
a 450-150-Ma interval (Figure 4).
(e) The boundary between Phanerozoic and Riphean, best
expressed on
the wavelet plane of reversal
frequency, is recognizable in all time series (Figure 4).
7. The general behavior of main parameters of the geomagnetic field in
the Neogaea (paleointensity and its
variations, and summary amplitude of variations in the field direction,
polarity, and reversal frequency)
provides a basis for the modeling of geomagnetic field generation and
characterizes processes in the core that
are comparable with processes in the lithosphere and at the Earth's surface.
This document was generated by TeXWeb
(Win32, v.1.0) on April 7, 1999.
 Periods of the integral wavelet spectrum
F(t) (15, 30, 50-60, 70, 100-110, 130, 180, 220, 280, and 390 Myr)
are essentially the same as those
previously obtained
[Marzocchi and Mulargia, 1992;
McElhinny, 1971;
Merrill and McElhinny, 1983;
Pechersky and Nechaeva, 1988].
As distinct from other methods of spectral analysis, wavelet data provide
time evolution of the spectrum. We consider the Neogaean
evolution of the wavelet spectrum (Figure 4)
[Galyagin et al., 1999].
First, the Vendian-Phanerozoic and Riphean patterns are drastically different.
Second, an oscillation with a period of about 400 Myr
is recognizable throughout the Vendian-Phanerozoic
interval; this period decreases from more than 450 Myr
in the Vendian to less than 400 Myr in the Late
Cenozoic. The lifetime of remaining oscillations with periods of 70 to 200 Myr
is much shorter. Thus,
oscillations with periods of 90 and 180 Myr
are traceable from 550 to 200 Ma, when both periods gradually
decreased from 200 to 170 Myr and from 100 to 90 Myr, respectively.
A 130-Myr period is stable and exists
from 180 Ma to the present time, and its resolution markedly improves
toward the Cenozoic; a 70-Myr period
exists from 160 Ma to the present time and decreases from 70 to 55 Myr.
Third, the Phanerozoic and Early
Riphean exhibit a series of "pulses" (approximately at 1650, 540, 370,
and 160 Ma) at the lower boundary of
the wavelet plane, which indicates their characteristic times to be about 30 Myr.
Periods of the integral wavelet spectrum
F(t) (15, 30, 50-60, 70, 100-110, 130, 180, 220, 280, and 390 Myr)
are essentially the same as those
previously obtained
[Marzocchi and Mulargia, 1992;
McElhinny, 1971;
Merrill and McElhinny, 1983;
Pechersky and Nechaeva, 1988].
As distinct from other methods of spectral analysis, wavelet data provide
time evolution of the spectrum. We consider the Neogaean
evolution of the wavelet spectrum (Figure 4)
[Galyagin et al., 1999].
First, the Vendian-Phanerozoic and Riphean patterns are drastically different.
Second, an oscillation with a period of about 400 Myr
is recognizable throughout the Vendian-Phanerozoic
interval; this period decreases from more than 450 Myr
in the Vendian to less than 400 Myr in the Late
Cenozoic. The lifetime of remaining oscillations with periods of 70 to 200 Myr
is much shorter. Thus,
oscillations with periods of 90 and 180 Myr
are traceable from 550 to 200 Ma, when both periods gradually
decreased from 200 to 170 Myr and from 100 to 90 Myr, respectively.
A 130-Myr period is stable and exists
from 180 Ma to the present time, and its resolution markedly improves
toward the Cenozoic; a 70-Myr period
exists from 160 Ma to the present time and decreases from 70 to 55 Myr.
Third, the Phanerozoic and Early
Riphean exhibit a series of "pulses" (approximately at 1650, 540, 370,
and 160 Ma) at the lower boundary of
the wavelet plane, which indicates their characteristic times to be about 30 Myr.
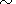 180 Myr) and a number of weak peaks
at 12, 23, 43, 60, 100, 180, 300, and 600 Myr
[Galyagin et al.,
180 Myr) and a number of weak peaks
at 12, 23, 43, 60, 100, 180, 300, and 600 Myr
[Galyagin et al.,  0.2.
Therefore, low-IPR
S means may be considered reliable,
which is especially important in the case of
Precambrian determinations dominated by IPR
0.2.
Therefore, low-IPR
S means may be considered reliable,
which is especially important in the case of
Precambrian determinations dominated by IPR

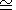 15o,
15o,  0.5
(RIPs are close to unity for many of those) mostly due to
numerous Thellier determinations from baked rocks, performed by
G. M. Solodovnikov and covering a 400-0-Ma interval.
RIP does not exceed 0.2 in the age intervals 70-80, 170-180, 220-230,
and 360-370 Ma; most
Riphean determinations have RIP = 0.4-0.5 mainly due to lacking
information about paleomagnetic
directions and inaccuracy of datings [Pechersky,
0.5
(RIPs are close to unity for many of those) mostly due to
numerous Thellier determinations from baked rocks, performed by
G. M. Solodovnikov and covering a 400-0-Ma interval.
RIP does not exceed 0.2 in the age intervals 70-80, 170-180, 220-230,
and 360-370 Ma; most
Riphean determinations have RIP = 0.4-0.5 mainly due to lacking
information about paleomagnetic
directions and inaccuracy of datings [Pechersky, 

 3.6)
3.6) 1022 A m
2 (RIP=0.65)
and
(8.5
1022 A m
2 (RIP=0.65)
and
(8.5 0.5-0.6
and
0.5-0.6
and